In The Diagram Which Must Be True For Point D To Be An Orthocenter

Of the points of concurrency the blue peg represents. Mark the picture to show which segments are congruent.

Triangle Centers Level 4 Challenges Practice Problems Online

Circumcenter incenter centroid or orthocenter.

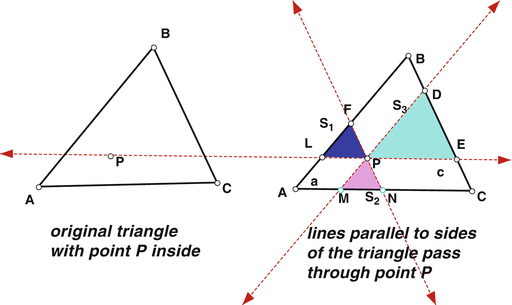
In the diagram which must be true for point d to be an orthocenter. In triangle abc the circumcenter and orthocenter are collinear with vertex a. I wanted to do a quick post on lsat logical reasoning must be true questions because people struggle a lot with these problems. 1 triangle abc must be an isosceles triangle.

You may want to make a different tracing for each center so your lines and arcs wont get confusingto determine. Point d is the intersection of three angle bisector. A m 1 m 3 b m 1 m 2 c m 1 m 2 d m 1 m 2.

In the diagram gb 2x 3. Which of the following statements must be true. Ag is the perpendicular bisector of bc.

As shown in the diagram points b and d are on different sides of line ac. Which classification of the triangle is correct. Be is the angle bisector of b.

2 triangle abc must be an equilateral triangle. Point p must be the 1 centroid 2 circumcenter 3 incenter 4 orthocenter 5 in the diagram below of abc cd is the bisector of bca ae is the bisector of cab and bg is drawn. 628721 to determine each point of concurrency you must perform their corresponding constructions.

Use the diagram of abc to the right. A copy of the diagram is shown below. Ag is the angle bisector of a.

Af cf and cd bd. Point d is also the intersection between three perpendicular bisector. 6 the diagram below shows the construction of the center of the circle circumscribed about abc.

In fact if there is common issue linking most people who arent happy with their scores on lsat practice tests its that they dont have a sufficient grasp of this question typeread more. He hd lh nh. Be is the perpendicular bisector of ac.

Cf is the angle bisector of c. Which is true about point d. Point d cannot be the orthocenter because the orthocenter of an obtuse triangle is located outside the triangle.

1 scalene triangle 2 isosceles triangle 3 equilateral triangle 4 right isosceles. Point h is the center of the circle that passes through points d e and f. Points d e and f are midpoints.

Triangle Coordinates Converter